An ellipse can informally be explained as an oval or a “squished” circle. It is defined as the set of all points in a plane such that the distance from two fixed points (foci) on the plane is constant. The major axis is the axis on which the foci lie; the longer axis of symmetry and the minor axis is the shorter axis of symmetry. The line that passes through the foci intersects the ellipse at two points which are called vertices. The chord that joins the vertices is the major axis, and its midpoint is called the centre of the ellipse. The chord that is perpendicular to the major axis at the centre is known as the minor axis.
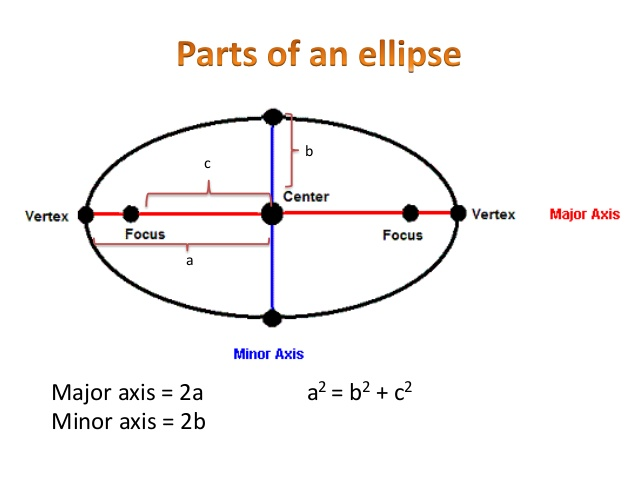
Figure: Parts of an ellipse.
Consider the graph below: The two foci for this ellipse are the two points lying on the horizontal axis that appear to be a little over 6 units from the origin. The origin is the centre of the ellipse. The distance from the centre to a focus is designated with a “c”.
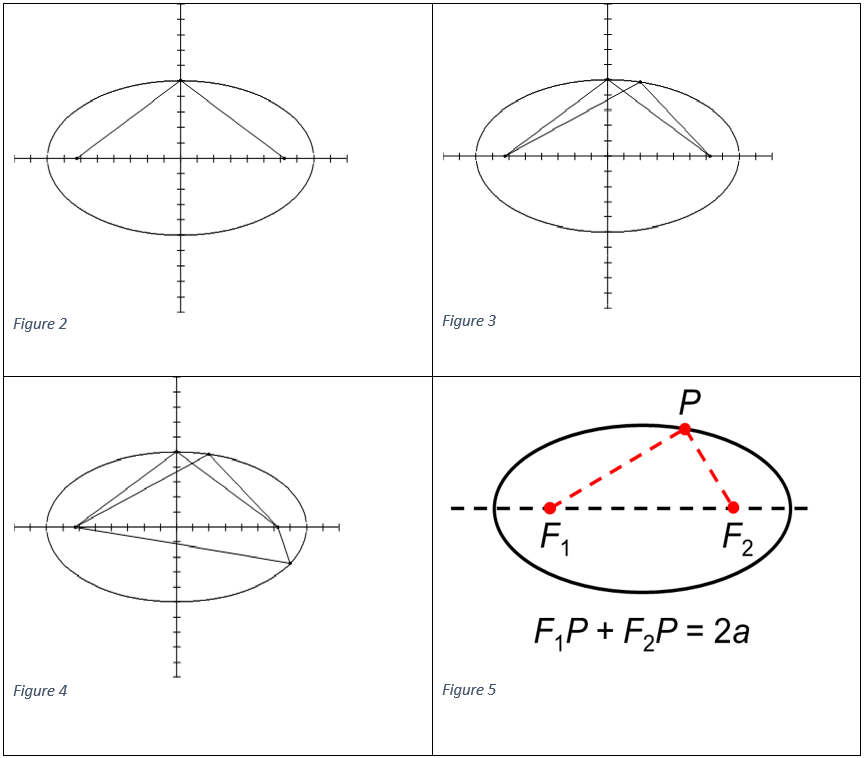
In figure 2, the segments drawn from the two foci to the point (0,5) on the ellipse are each 8 units in length. Their total length is 16 units. This total length is also the length of the major axis. Two more segments are added in figure 3, drawn from the foci to the point (2,4.84) on the ellipse. Their lengths are 9.556 and 6.434. The sum of these lengths is again 16 units. In figure 4, The two latest segments, drawn to the point (7,-2.42) on the ellipse, are 13.463 units and 2.537 units in length, a sum of 16 units.
The sum of the distance of a point on the ellipse from two fixed points is always higher than the distance between two points. This means that P1F1 +P1F2 = P2F1 +P2F2 = 2a as shown in figure 5.
Now, let’s see the graph below:
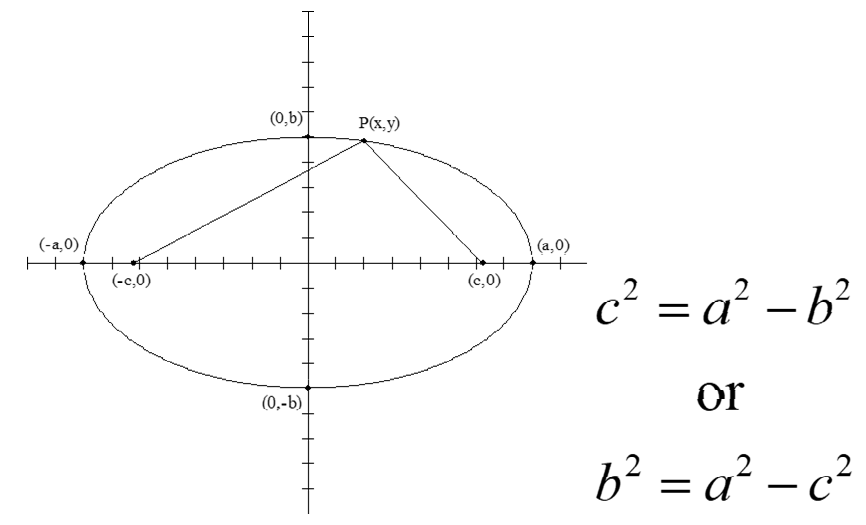
Figure 6
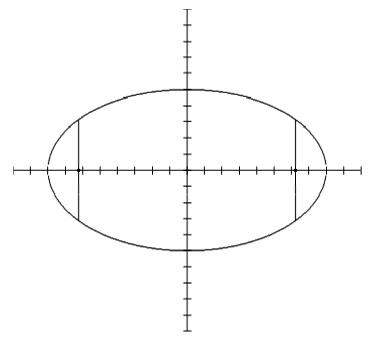
The ends of the major axis are located at (a,0) and (-a,0).The ends of the minor axis are at (0,b) and (0,-b).The foci are at (c,0) and (-c,0).The sum of the distances from point P to the foci is 2a.A chord through a focus and perpendicular to the major axis is called a latus rectum.The major axis is equal to 2a and the minor axis is equal to 2b. The endpoints of the two latus recti are found using the equivalence: c2 = a2 – b2 which provides the endpoints ($\pm c, \frac{b^{2}}{a}$)
Figure 7: Latus rectum
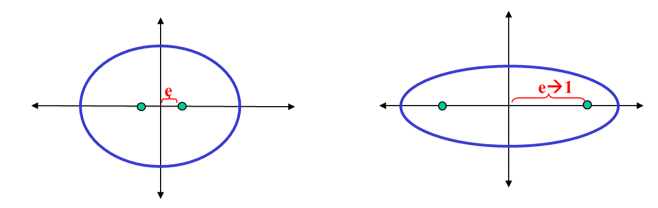
To measure the ovalness of an ellipse, we use the concept of eccentricity. The eccentricity ‘e’ of an ellipse can be explained by the ratio e = c/a where 0 < e < 1 is valid for every ellipse.