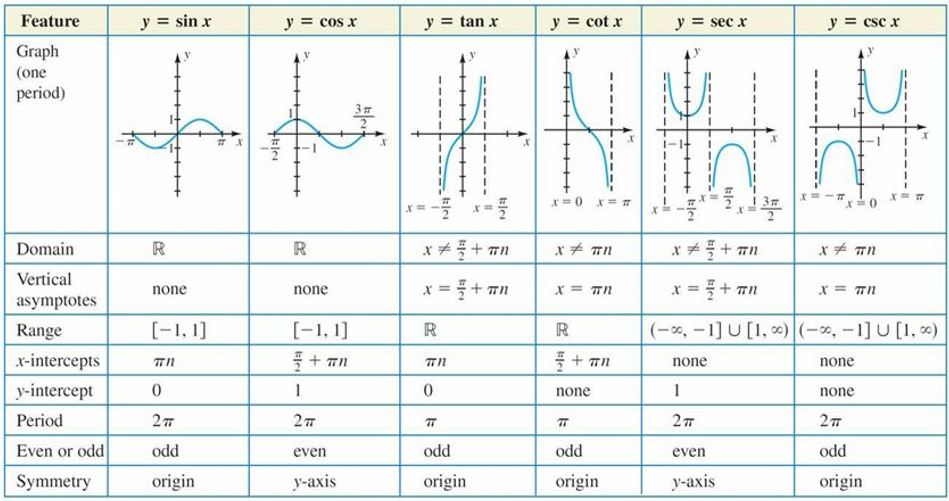
Function’s domain is defined as the particular set values that an independent variable contained in a function can accept the work. The range exists as resulting values which a dependent variable can hold a value of ‘x’ changes all through the domain.
For Cosine and Sine Functions, the Range and Domain
There are no limitations on cosine and sine’s domain functions. So, their domain results in the form of x ∈ R.
It’s important to note that, nonetheless, the range for y = cos (x) and y = sin (x) is between the range of (-1 & 1). Hence, the changes found in these functions regarding stretches and shifts will result in affecting the range of trigonometric functions but not the domain of the trigonometric functions.
Domain and Range of Sine Function
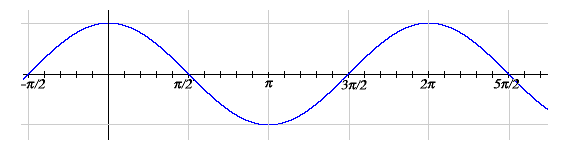
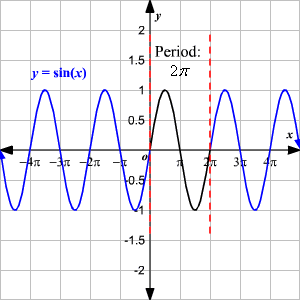
y = f(x)= Sin(x)
Range: The value lies between -1 ≤ y ≤ 1
Domain: It’s determined for all the ‘x’ real values
Period: 2π = 360º
Sine Function is an odd function
The Graph of sin(x) function:
Domain and Range of Cosine Function
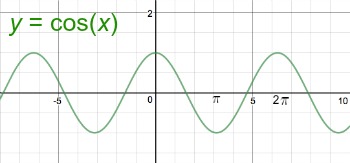
y= f(x) = cos(x)
Range: the value lies between -1 ≤ y ≤ 1
Domain: Defined for all the x real values
Period: 2π
Cosine is an even function
The Graph of cos(x) function:
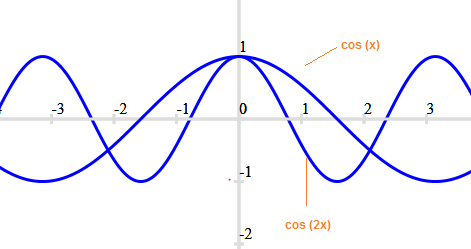
From the above graph, we can see that the range remains there and graph reduces
Domain and Range for Tangent functions
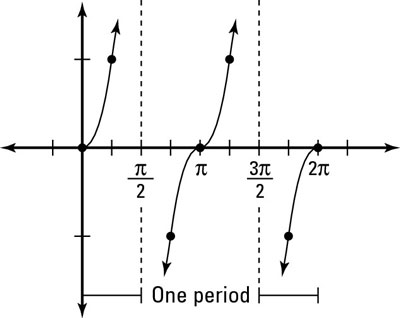
Note that the function y = tan (x) consist of vertical asymptotes at ![]() . Hence,
. Hence,
For – y=f(x)=tan(x)
Range: All real numbers (or y ∈ R)
Tangent’s Domain: Defined for all x real values, except x ≠(2n + 1)(π/2), where n is any integer.
Period: π
Tangent is an odd function
As a result. from the above domain and range, changes will affect range but will affect the domain.
The Graph of tan(x) function
Domain and Range For Cotangent Function
y=f(x)=cot(x)
Range: All the real numbers
Domain: Defined for all the x real values; except x ≠nπ, where n is any value of an integer
Period: π
Cotangent is an odd function
The Graph of cot(x) function
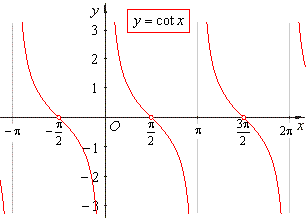
Domain and Range For Secant Function
y=f(x)=sec(x)
Range : (-∞,-1] ∪ [1,∞)
Domain: It is defined for all real values of x except x ≠(2n + 1)(π/2) where n is any value of the integer
Period: 2π
Secant is an even function
The Graph of sec(x) function
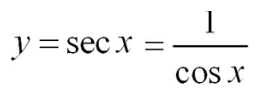
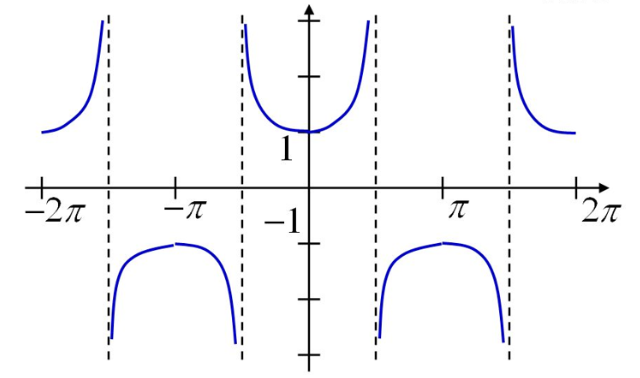
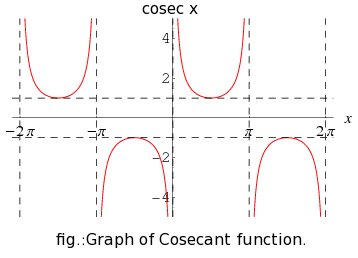
Domain and Range For Cosecant Function
y=f(x)=cosec(x)
Range: (-∞,-1] ∪ [1,∞)
Domain: Defined for all x real values; except x ≠nπ, where n exist as any integer value
Period: 2π
Cosecant is an odd function
The Graph of cosec(x) function
Summary of all trigonometric functions of Real Numbers and their graphs: