Recall from previous chapters that inverse of a function f can be written as f-1, where the expression sin-1 is read as “the inverse sine.” In this notation,-1 indicates the inverse and not the reciprocal of the sine function.Other names for inverse trigonometric functions are arcsine, arccosine, and arctangent functions. The inverses are not functions themselves because there are multiple values of θ for a particular value of a. For example, suppose that you want to find cos-1. Based on the unit circle, angles that measure and radians and other co-terminals all have a cosine of1/2.
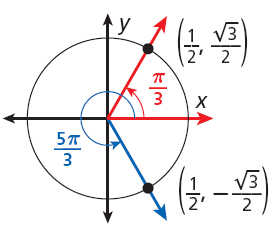
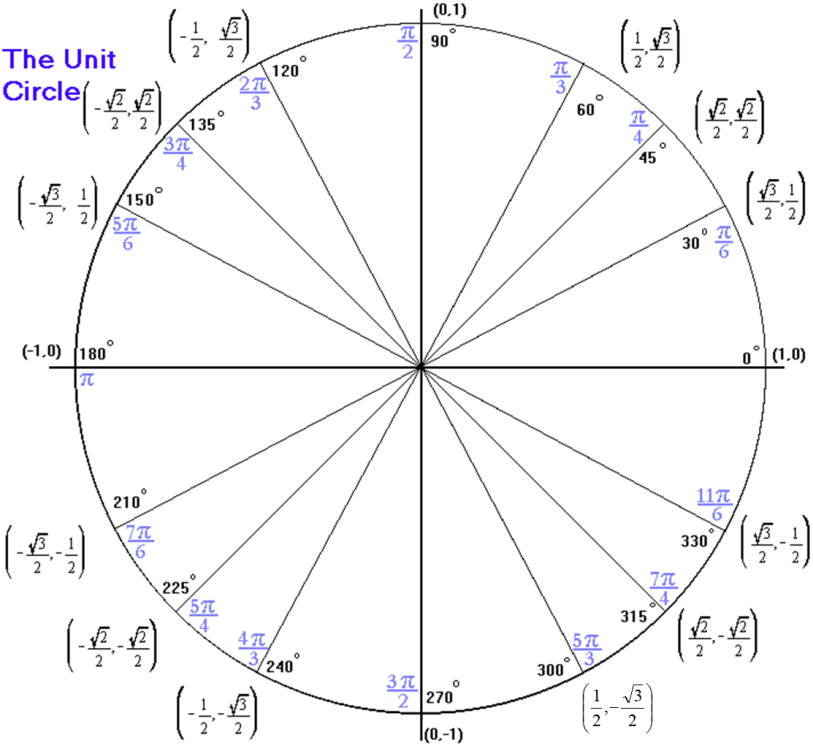
Example: Find all possible values of the following function: cos-1
First, use the unit circle to get the values between 0 and 2 radians for which cos θis equal to Use the x-coordinates of points on the unit circle to begin the deduction.
![]()
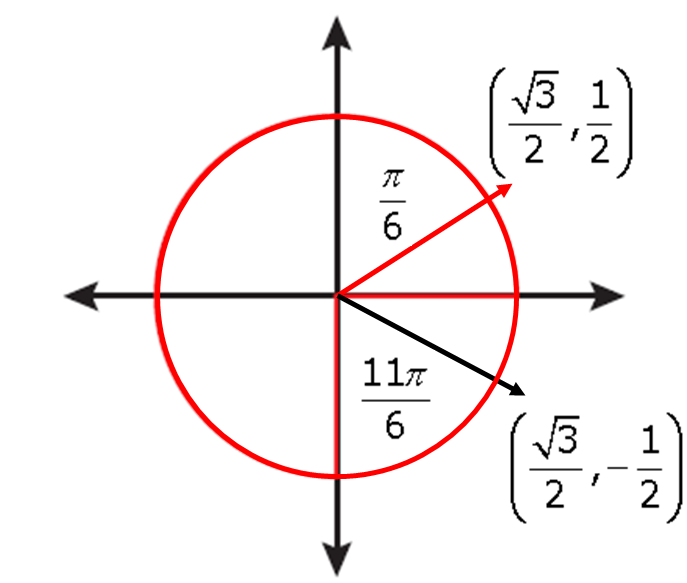
Find the angles that are coterminal with angles measuring and radians and also add integer multiples of 2π radians as shown below. Here, n is an integer:
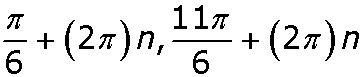
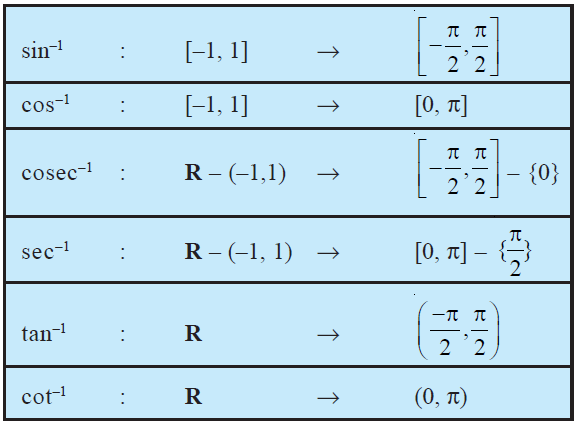
There are more than one value of θproduces the same output value for a given trigonometric function. Therefore, it is necessary to restrict the domain of each trigonometric function in order to define the inverse trigonometric functions.The trigonometric functions with restricted domains are indicated with a capital letter. The domains and range of the Sine, Cosine, and Tangent functions are restricted as follows.

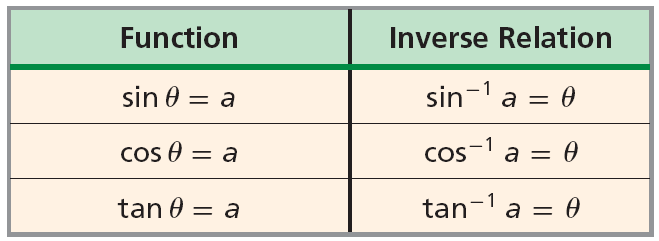
Recall that the angle is the input for a trigonometric function, while the ratio is its output. For the inverse trigonometric functions, it is the opposite. A unit circle may be used to estimate values of angles from the domain and range of the functions.
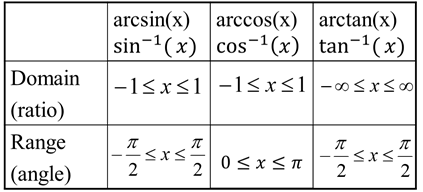
For each value of x in the domain of the inverse trigonometric functions, there is only one value of θ. Therefore, while tan-1 has many values, tan-11 has one value only.The principal Solutions to Arccoslies between 0° and 180° or 0 and π radians. This includes Quadrant II angles if the number is negative and Quadrant I angles if the number is positive.Alternatively, principal Solutions to Arctan lies between -90° and 90° or -π/2 and π/2 radians. The range includes Quadrant IV angles if the number is negative and Quadrant I angles if the number is positive. The following table provides the principal value branches for the trigonometric functions: