Set of all points in a plane at a fixed distance (radius) from a fixed point (centre). Let the radius of a circle be r and the centre be (h, k) and suppose point P(x, y) be any point on the circle. This means that the distance between (x, y) and (h, k) is r.By distance formula we can obtain:
$r=\sqrt{(x-h)^{2}+(y-k)^{2}}$
$r^{2}=(x-h)^{2}+(y-k)^{2}$
Square both the sides to obtain the general form of a circle to deduce centre-radius form:
$(x-h)^{2}+(y-k)^{2} =r^{2}$
the centre is at (h, k) and the radius is r (notice that in the equation r is squared)
If the centre is at the (0, 0), then the standard equation is $(x)^{2}+(y)^{2} =r^{2}$
Where the square root of both sides of an equation, remember that you must include the +ive and -ive roots.
The general form of a circle is $x^{2}+y^{2}+Dx+Ey+F=0$
Following are the steps to convert General Form to Standard form:
- Move the x terms together and the y terms together.
- Move C to the other side.
- Complete the square for x and y.
- Factor and simplify both the equations.
In completing the square if:
$r^{2}>0$ circle
$r^{2}=0$ degenerate circle / point circle
$r^{2}<0$ the empty set (not possible)
Example 1. Give the center and the radius of this equation: (x – 1)$^{2}$ + (y + 3)$^{2}$ = 9
center = (1, -3)
radius = 3
Example 2. Give the centre and the radius of this equation: $x^{2}+y^{2}+8x-10y=23$
Complete the square and add (b/2)$^{2}$ to both sides of the equation:
$(x^{2}+8x+16)+(y^{2}-10y+25)=23+16 +25$
$(x+4)^{2}+(y-5)^{2}=64$
$C(-4, 5);r=8$
Example 3. Determine an equation of a circle congruent to the graph of $x^{2}+y^{2}=16$ and translated 3 units right and 1 unit down.
$(x-3)^{2}+(y+1)^{2}=16$
Example 4. Determine what $3x^{2}+3y^{2}-30x+18y+178=0$ represents.
$3(x^{2}-10x+25)+3(y^{2}+6y+9)=-178+75+27$
$3(x-5)^{2}+3(y+3)^{2}=-76$
$(x-5)^{2}+(y+3)^{2}=-\frac{76}{3}$
Since $r^{2}<0$, the equation represents an empty set.
Example 5. Determine an equation of a circle that satisfies the centre at (2, 3) tangent to line $5x+6y=14$
Distance from point to a line:
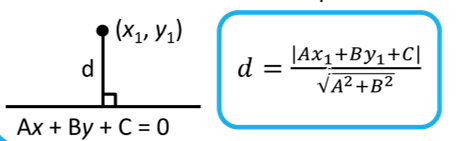
$r=\frac{\vert 5(2)+6(3)-14\vert }{\sqrt{(5)^{2}+(6)^{2}}}=\frac{\vert 14\vert }{\sqrt{61}}$
$(x-2)^{2}+(y-3)^{2}=\frac{196}{61}$
Example 6. Find the centre, the length of the radius, and write the equation of the circle if the endpoints of a diameter are (-8,2) and (2,0).
Centre: Use the midpoint formula:
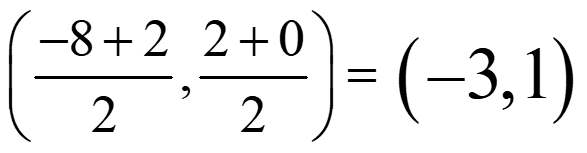
Radius: use distance formula with radius and an endpoint:
![]()
Substitute the values for centre and radius in the standard equation:
![]()