Coulomb’s law is a quantitative statement about the force between two point charges and it is an analog of Newton’s Universal Law of Gravitation. Coulomb’s law states that the force of attraction or repulsion between two stationary point charges is directly proportional to the product of the magnitude of two charges and inversely proportional to the square of the distance between them.
Figure:1.a
If $q_{1}$and $q_{2}$are two point charges separated by a distance $r$, then according to Coulomb’s law, the force $F$ of attraction or repulsion is between them,
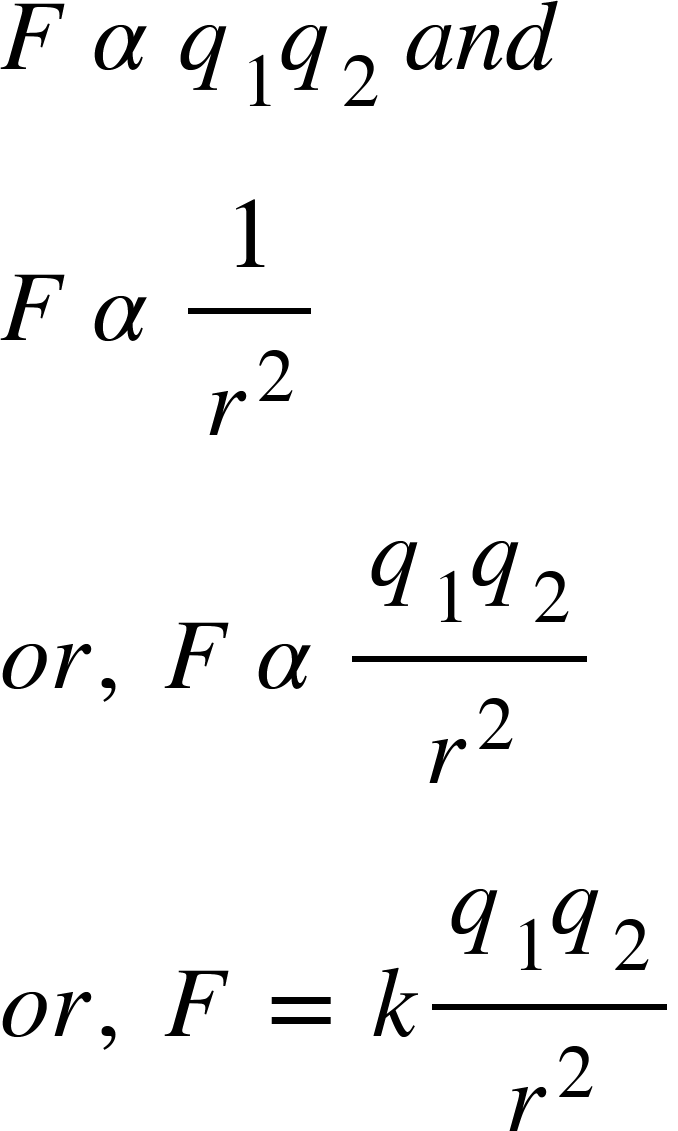…………………(1)
Where $k$ is a constant of proportionality.
The value of $k$ depends on the nature of the medium between two charges and the system of units we choose to measure.
In SI units system, when two charges are in vacuum or air, the value of $k$ is given by,
Where $\epsilon _{0}$is the absolute permittivity of free space and its value is,
So, from equation (1), we get,
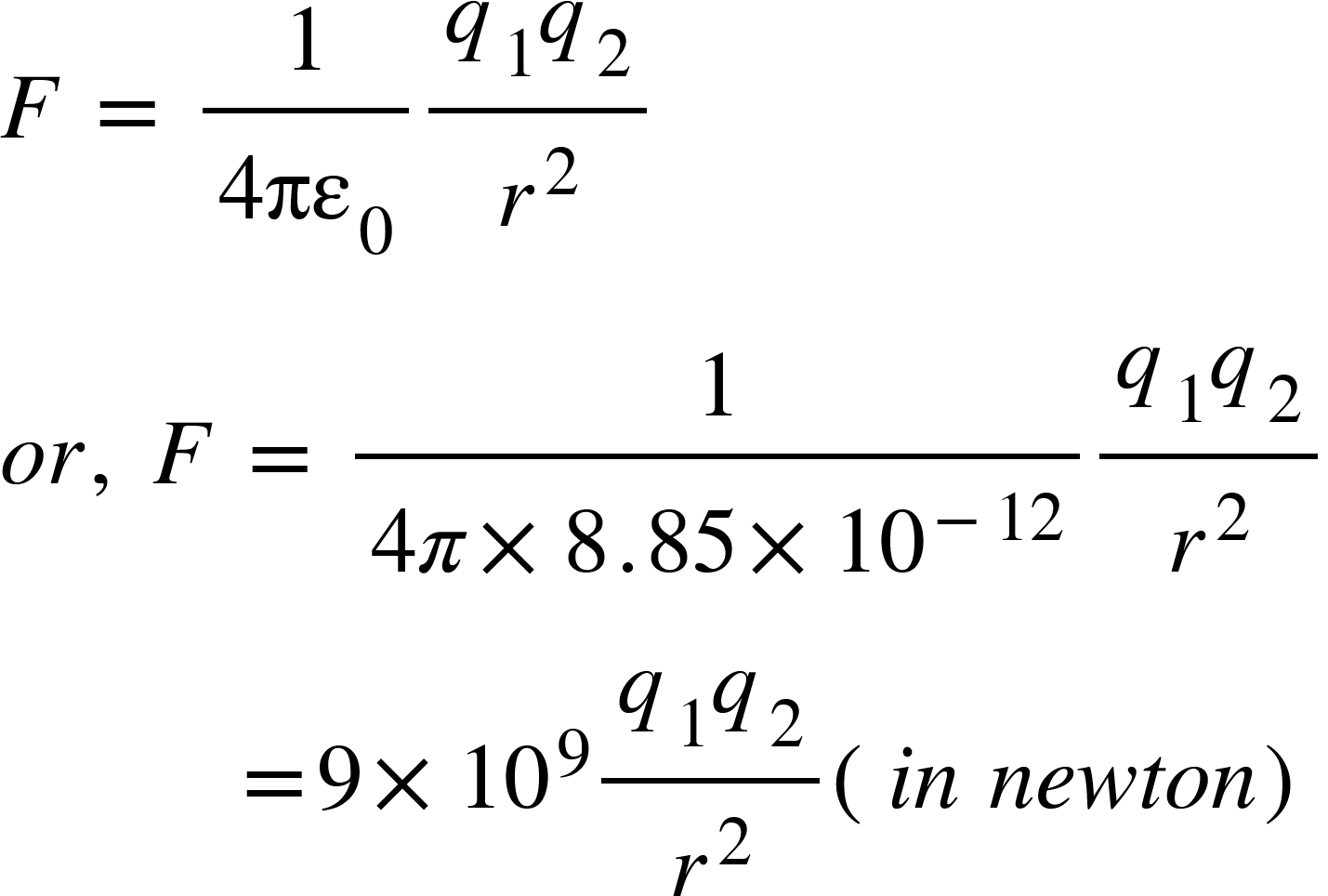
if the charges are in a medium (example – water etc.) other than air and vacuum then force between these two charges is given by,
Where $\epsilon $is a constant and is the permittivity of the medium in which charges are present.
Points to remember:
- The direction of Coulomb’s force is along the line joining two charges. It can be inward or outward depending on attraction or repulsion between the charges. We will discuss vector form of Coulomb’s law later.
- Coulomb’s Law holds for two or more point charges which are rest.
The SI unit of electric charge is Coulomb.
If $q_{1}$= $q_{2}$= $1C$and $r=1m$, we get,
We can define Coulomb as one Coulomb is the amount of charge that repels an equal and similar charge with a force of 
when placed in a vacuum or air at a distance of one meter from it.
Relative permittivity:
Force between two charges in a medium other than vacuum or air is given by,
And, force between two charges in vacuum or air is given by,
Now,
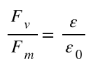
Or
$\varepsilon _{r}$is called the relative permittivity or dielectric constant $(k)$of the given medium.
Therefore, when two charges are placed in a material medium of dielectric constant $k$
the force between them $1/k$ times the force in a vacuum.
Coulomb’s law in vector form:
To express Coulomb’s law in vector form, let us consider a diagram given below.
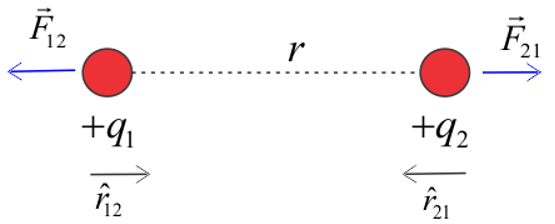
Figure:1.b
We have two positive charges $q_{1}$ and $q_{2}$ separated by a distance $r$. As they are like charges, they both would repel each other. When $q_{1}$exerts a force on charge on $q_{2}$, the charge $q_{2}$also exerts an equal and opposite force (according to Newton’s third law of motion) on charge $q_{1}$
So, force on charge $q_{2}$due to charge $q_{1}$can be written as,
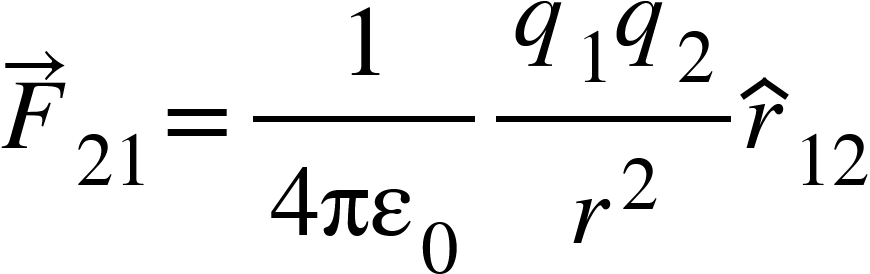
Where 
is the unit vector in direction from $q_{1}$ to $q_{2}$.
Similarly,
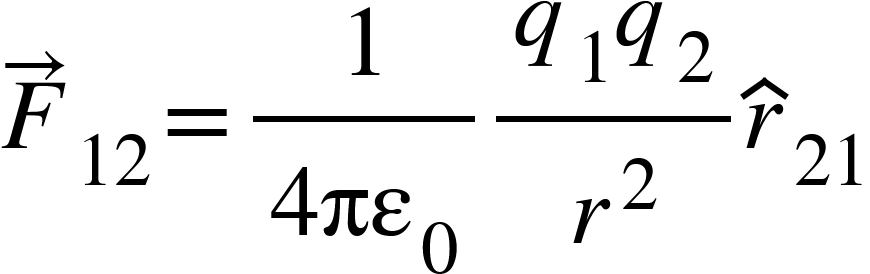
Where 
is the unit vector in direction from $q_{2}$to $q_{1}$.
Similarly, for unlike charges, the diagram is shown below.
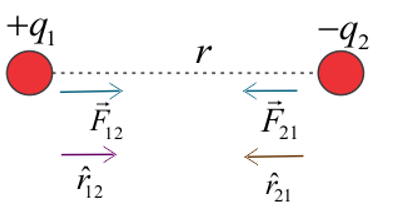
Figure:1.c
Points to remember:
- Coulomb force acts along a line joining the center of two charges. So it is an example of central force.