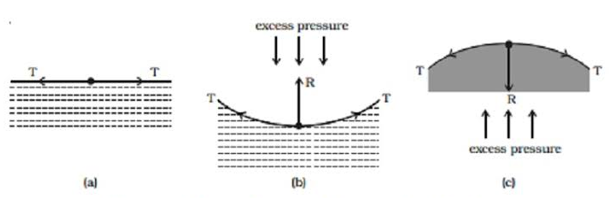
One common manifestation of surface tension is the difference in pressure it causes across a curved surface. For simplicity, we consider first a liquid surface that is curved in only one plane but is flat in a direction perpendicular to that plane. The pressures in the different fluids on either side of this interface are denoted by pO (on the outside of the curve) and pI (on the inside of the curve). Now consider all the forces acting on a small section of the surface of length and unit dimension which is normal.
Consider all the forces in the direction normal to the center of the fluid element. By the result described in static forces the net force in the outward direction due to forces near and at a fluid surface, pO and pI will be 2R sin dθ(pI − pO). Opposing this will be the surface tension components that are known to force S acting on the two ends of the section of surface which yield a force from the core, equal to 2 sin dθS. Thus the surface tension causes greater pressure inside the surface and the difference is the surface tension divided by the radius of curvature (so that a flat surface yields no pressure difference). Note that it makes no difference whether the liquid is on the outside or on the inside.
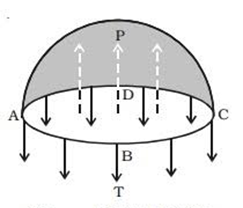
Now consider a spherical surface, specifically the spherical drop. If the drop is cut in half the force imposed by surface tension on the half which is remaining will be 2πRS.
Opposing that will be the pressure difference (pI − pO) acting on the projected area πR2 and therefore, in equilibrium,2πRS = πR2(pI − pO) (Cm3).
A more suitable way to visualize this is that, on the outer surface, the curvatures from every 2 perpendicular directions contribute equally to the pressure difference, which is caused by the surface tension. Indeed, a general three-dimensional surface will contain two principal radii of curvature, R1 and R2, and it can be easily presented that the resulting pressure difference in this general case is given by pI − pO = S – 1 R1 + 1 R2 (Cm5).
In the special case of the cylindrical surfaces, R1= R and R2 = ∞ and in the special case of the sphere,R1 = R2 = R.