Magnetic Field Intensity Due to AMagnetic Dipole (bar magnet) Along its Axis and Perpendicular to its Axis
In physics the magnetic field intensity is also called as magnetic field strength. The magnetic field is created by the current-carrying wires. According to the law of Biot-Sarvat, the magnetic field at any point due to the element of the conductor is carrying current, that is directly proportional to the strength of the current, length of the element, and to the Sine of angle that is between the line of joining of element to the point and element. But it is inversely proportional to the square of the distance, that is between the point and element.

At the point O, the magnetic field is dB, that can be stated as below.
![]()
Then
![]()
Where the next situation can be stated as follows.
![]()
Here K is the constant of proportionality. The value stated below represents the permeability of free space.
![]()
In the vector form, the entire process can be represented as below.

Let consider a circular coil that is having radius r, and is carrying the current I. There is a point p that is lying at a specific distance from the center of the coil. It can be considered that the loop is having a large number of shorter elements which are generating smaller magnetic fields. The total field that is experienced by point p, is the sum of total contributions from all elements. The field is uniform at the center of the coil. With the increase in the location of a point from the center of the coil, a decrease in the field is observed.
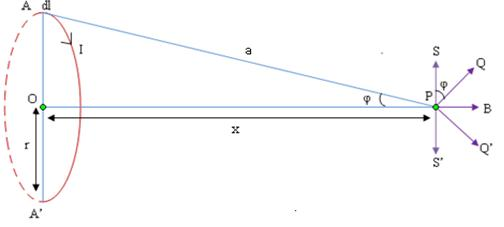
According to Biot-Savart’s law, the field arising due to smaller elements of the circle is positioned at A, and it can be represented as follows.

It is possible to resolve the area into two components one along the PS and other on OP. The component OP is along the axis, whereas, PS is perpendicular to OP. Due to perpendicular component, the PS is exactly canceled, and the field due to current is centered at point A. The total magnetic field at the point, that is at the distance x from the axis of a coil having the radius r can be represented as follows.
![]()
If n is the number of turns in coil then
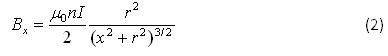
As the field from the coil is perpendicular to horizontal intensity then the needle of the compass is aligned at the angle, along with vector sum of the fields. The horizontal component of the field is greatly variable over the surface and this variation also exists along the axis.