Introduction to Number of Atoms in a Unit Cell

According to our knowledge, there are several unit cells in a crystal lattice. In the unit cell, each of the constituent particle comprising of a molecule, ion, or atom carries a fixed and specific position, known as the lattice site.

We can easily do the calculation of the number of molecules/atoms and ions in the unit cell by examining and determining constituent particles’ position and nature in the unit cells.

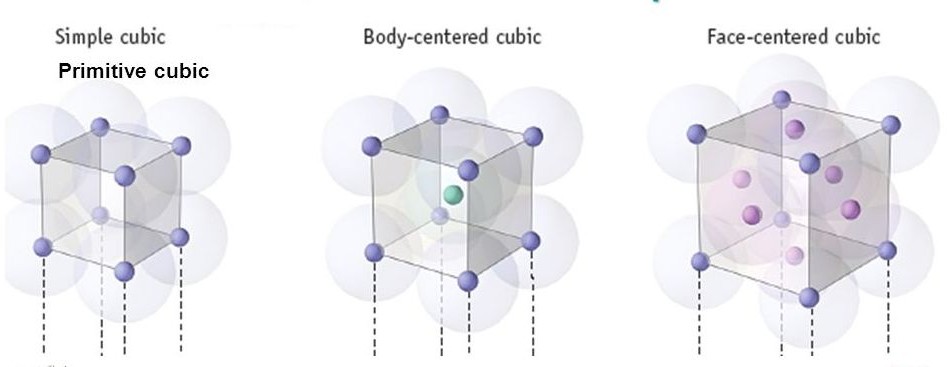
Fig 1: Types of Unit Cell

Primitive Cubic Unit Cell

In Primitive Cubic Unit Cell, the atoms are located only at the corners. This means that 8 atoms are placed on the lattice at 8 corners. Located at the corner, each atom contributes about 1/8 of the original cell’s volume. Therefore, since there’s a net sum of eight (8) atoms present in the primitive cubic unit cell, the primitive cubic unit cell’s total number of atoms is given by:

Thus, there’s 1 atom only present in the primitive cubic unit cell.

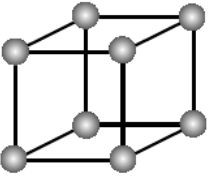
Fig 2: The corners acquires 1/8th part only of the atom

 

Body- Centred Cubic Unit Cell

In a Body-Centred Unit Cell, at 8 corners, 8 atoms are placed and 1 atom is located at structure’s centre. Thus, Body-Centred Unit Cell’s total atoms is given by:

Seeing that, on the corners, 8 atoms are present and each atom contributes 1/8 of the original cell’s volume. Accordingly, the total atoms of Body-Centred Unit Cell will be:

There are two corners present; one having 8 corners and another is sharing 1/8th volume of the whole cell, so

In addition, the atom located at the centre is entirely present at the cell’s centre and which cannot be shared.

1 × 1 = 1 atom

Thus, in total, there’re two atoms in the Body-Centred Unit Cell.

Fig 3: Atoms in Body-Centred Unit Cell

Face-Centred Cubic Unit Cell

The face-centred cubic unit cell is having atoms that are present at the centre of all faces and at 8 corners. Additionally, each atom placed on unit cell’s centre is being shared by 2 adjoining unit cells. Thus, half of the atoms only refer to a Single Unit Cell.

Therefore, in Face-Centred Cubic Unit Cell

At 8 corners, 8 atoms are placed, thus, each corner will gain 1/8 part of an atom

With six faces present, each face gains 1/2 part of the atom, then

The net sum of atoms in a Face-Centred Unit Cell = 1 + 3 = 4 atoms

End-Centred Cubic Unit Cell

In the End-Centred Cubic Unit Cell, in a cube, on the 8 corners, 8 atoms are present. One atom each is having its presence on cube’s 2 opposite faces.

Thus, in the End-Centred Cubic Unit Cell,

On 8 corners, 8 atoms present, as a result, each of the atoms contributes a 1/8th cells’ part.

At the cell’s centre, two atoms are present. Each atom is making a contribution of 1/2th cell’s portion/part.

The total of atoms located in the End-Centred Cubic Unit Cell = 1 + 1 = 2 atoms

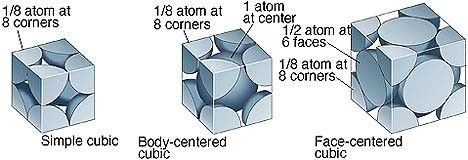
Fig 4: The Contribution of Atom in various unit cells

Given below is the table representing the total number of atoms in a unit cell, in a summarized form

Type of Unit Cell	Number of atoms at corners	Number of atoms on faces	Number of atoms in center	Total
Simple Cubic	8 × 1/8 = 1	0	0	1
Body Centred Cubic	8 × 1/8 = 1	0	1	2
Face Centred Cubic	8 × 1/8 = 1	6 × 1/2 = 3	0	4
End Centred Cubic	8 × 1/8 = 1	2 × 1/2 = 1	0	2

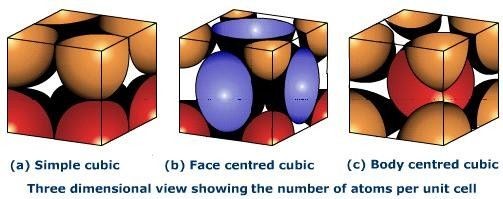
Fig 5: Types of Unit Cell