Probability is a number of ways a certain event can occur to the number of possible outcomes. An outcome is one possible result of a probability and the act that leads to a single outcome that cannot be predicted with certainty is called an experiment. For instance, today there is a 45% chance of rain and the odds of winning the lottery are a million to one.
The random experiment is a process or activity which produces a number of possible outcomes. The outcomes of a random experiment cannot be predicted with utmost certainty. Random outcomes occur in an irregular manner if each outcome is equally likely to occur. A probability helps in understanding a quantitative description of the likelihoods associated with several outcomes.
This means that an experiment is called a random experiment under two conditions:
- The experiment has more than one possible outcome.
- Prediction of the outcome is not possible in advance.
The exact outcome is unknown before conducting the experiment. All possible outcomes of the experiment are known and each outcome is equally likely. The experiment can be repeated under uniform conditions. Together these conditions produce regularities or patterns in outcomes. Statistical regularity can be seen when random outcomes are repeated for a long period of time.
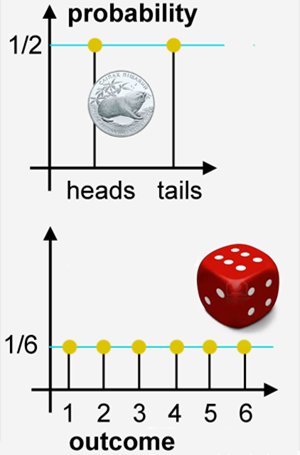
Example: Flip of a coin: two outcomes: heads or tails
We are unable to predict each toss whether is Head or Tail. After several trials, a statistical pattern can be observed and we can predict that 50% of the time heads will occur and 50% of the time tails will occur.
Example: Rolling a die – outcomes
![]()
We are unable to predict the outcome but in the long run can one can determine that each outcome will occur 1/6 of the time. Hence, we can use symmetry to understand the possible outcome. Each side is the same. One side must not happen more frequently than another side in the long run. If the die used for the experiment is not balanced then these conclusions may not be true.
$Theoretical probability=\frac{Number of favourable outcomes}{Total number of outcomes}$
A favorable outcome is an outcome for a specified event. Probability is a number from 0 to 1, including 0 and 1, and can be expressed as a decimal, fraction or percent. The probability of A is written as P(A). The sum of the probability of all the outcomes in a sample space is 1.